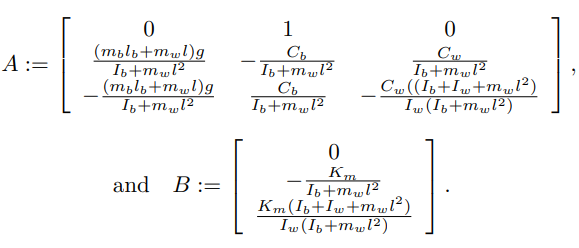Constant Offset matrix

Question

I've been reading about controlling systems via momentum wheels.

Such as in Satellites, or fancy inverse pendulums.

In a particular paper, the writer tries to explain away an offset and settling of the system angle and reaction wheel angular velocity at non-demand conditions because of the dynamics of the control scheme will give to a constant motor angular velocity when there is a constant offset. Given the matricies A and B and the Kd gains aquired from the LQR method from a SSM such as:

$A=\left( \begin{array}{ccc} 0 & 1 & 0 \\ \frac{g \left(l_b m_b+l m_w\right)}{\Theta _b+l^2 m_w} & -\frac{R_b}{\Theta _b+l^2 m_w} & \frac{R_w}{\Theta _b+l^2 m_w} \\ -\frac{g \left(l_b m_b+l m_w\right)}{\Theta _b+l^2 m_w} & \frac{R_b}{\Theta _b+l^2 m_w} & \frac{R_w \left(\Theta _b+l^2 m_w+\Theta _w\right)}{\Theta _w \left(\Theta _b+l^2 m_w\right)} \\ \end{array} \right)$

$B=\left( \begin{array}{c} 0 \\ -\frac{\text{Km}}{\Theta _b+l^2 m_w} \\ \frac{R_w \left(\Theta _b+l^2 m_w+\Theta _w\right)}{\Theta _w \left(\Theta _b+l^2 m_w\right)} \\ \end{array} \right)$

$Kd=\left( \begin{array}{c} \text{K1} \\ \text{K2} \\ \text{K3} \\ \end{array} \right)$

They explain

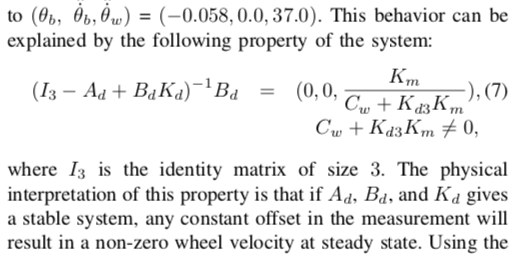
As it can be seen from the plot, the estimated state of the system converges to ($\phi$b , $\phi'$b , $\phi'$w ) = (−0.058, 0.0, 37.0). This behavior can be explained by the following property of the system:

$$V=(\text{IdentityMatrix}[3]-A+B.\text{Kd})^{-1}.B=\left( \begin{array}{c} 0 \\ 0 \\ \frac{\text{Km}}{\text{K3} \text{Km}+R_w} \\ \end{array} \right)$$

However, when working out their maths...I get anything but their solution, where the first two components or $V$ are equal to 0.

Is this particular equation a known one assumed to give zeros in the first two components? Or is there a mistake of some kind? Unfortunately there are no references given to their assumption.

The exact text for context:

Answer 1

I actually spent a while trying to figure this out, and I realized that they're talking about Final value theorem.

Here's a paper (warning: PDF) that gives the equation you've provided in your paper with a correction:

$$ \left(sI - \left(A-BK\right)\right)^{-1}B \\ $$

Note the Laplace operator $s$! The final value theorem says that you can get $\lim_{t\rightarrow\infty} x(t)$ by taking the limit $\lim_{s\rightarrow0}sX(s)$.

Now, I couldn't get the right answer with the matrices you provided, but I think I found your source material ("The Cubli: A Cube that can Jump Up and Balance", Gajamohan et al 2012), which gives:

When I use these values and use $Kd = [k1, k2, k3]$ (Note that this is a row vector, not column vector as in your question), I get the following:

clear all;
clc;
syms L Lb mw mb g Km K1 K2 K3 Ib Iw Cb Cw;
syms s;
A = [0, 1, 0; ...
    (mb*Lb+mw*L)*g/(Ib+mw*L*L), -Cb/(Ib+mw*L*L), Cw/(Ib+mw*L*L); ...
    -(mb*Lb+mw*L)*g/(Ib+mw*L*L), Cb/(Ib+mw*L*L), -Cw*(Ib+Iw+mw*L*L)/(Iw*(Ib+mw*L*L))];
B = [0; ...
    -Km/(Ib+mw*L*L); ...
    Km*(Ib+Iw+mw*L*L)/(Iw*(Ib+mw*L*L))];
Kd = [K1, K2, K3];

eqn = ((s*eye(3) - (A-B*Kd))^(-1))*B;

solution = limit(eqn, s, 0);

simplify(expand(solution))

ans =

               0
               0
 Km/(Cw + K3*Km)

Hope this helps!!