DH parameters for a PPP arm

Question

I found this picture on the web and was working through it to make sure I understood the derivation for a PPP arm. I believe for link 2, alpha_2 ought to be -90 and not +90.

Additionally, I don't understand why theta_3 is -90, give that link_3 is only a translation of link_2.

Am I misunderstanding something or are the provided DH parameters incorrect with respect to the provided drawing?

Answer 1

The DH parameters comes from the common normal between two consecutive Z axes. Every time you're in doubt about the DH parameters, you can follow common guidelines between two consecutive axes.

In this case, $d_i$ is the distance along previous Z starting from the previous origin O to the intersection of the new X and previous Z axis, which is variable in the case of prismatic joints.

$r_i$ (or $a_i$) is the distance in current X starting from current origin O to the intersection between previous Z and current X.

$\theta_i$ is the angle in previous Z to align the previous X with the new X.

$\alpha_i$ is the angle in current X to align previous Z to current Z.

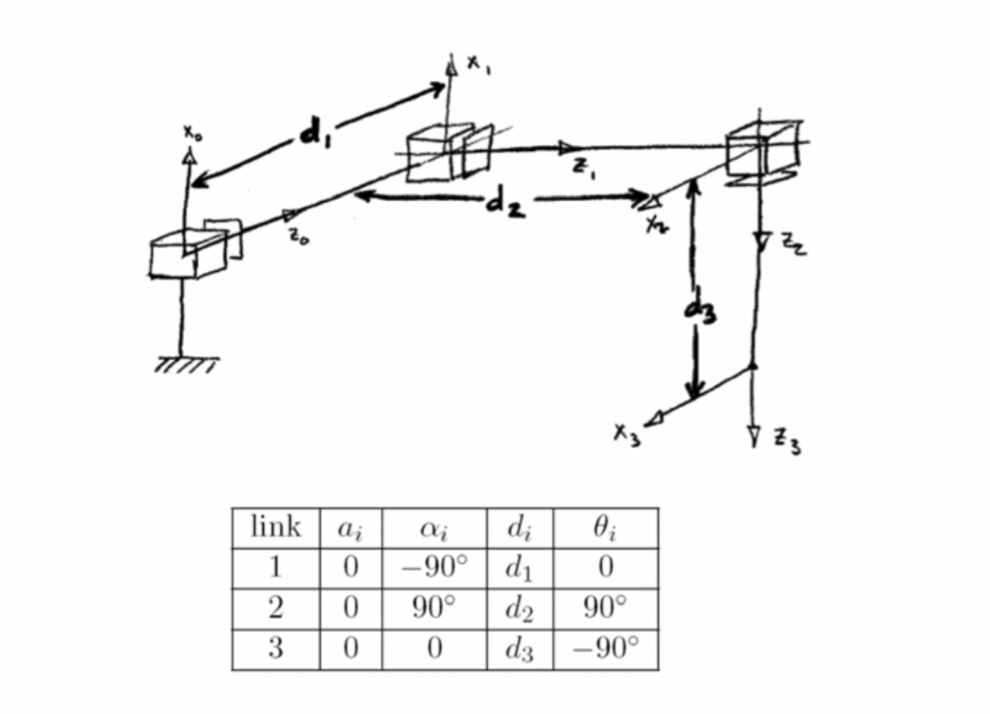
From the picture, starting with link 1:

• $d$: The distance from previous O to the intersection of previous Z and current X is variable, so it's d1.

• $a$: The distance in current X starting from current O to the intersection between previous Z and current X is 0.

• $\theta$: The angle in previous Z to align previous X with the new X is 0.

• $\alpha$: The angle in current X to align previous Z to current Z is -90 (right hand rule).

For link 2:

• $d$: The distance from previous O to the intersection of previous Z and current X is variable, so it's d2.

• $a$: The distance in current X starting from current O to the intersection between previous Z and current X is 0.

• $\theta$: The angle in previous Z to align previous X with the new X is 90 (right hand rule).

• $\alpha$: The angle in current X to align previous Z to current Z is -90 (right hand rule).

For link 3:

• $d$: The distance from previous O to the intersection of previous Z and current X is variable, so it's d3.

• $a$: The distance in current X starting from current O to the intersection between previous Z and current X is 0.

• $\theta$: The angle in previous Z to align previous X with the new X is 0 (right hand rule).

• $\alpha$: The angle in current X to align previous Z to current Z is 0 (right hand rule).

So the correct DH Table for this robot would be:

---

Link 1: $a=0$, $\alpha=-90$, $d=d1$, $\theta=0$

---

Link 2: $a=0$, $\alpha=-90$, $d=d2$, $\theta=90$

---

Link 3: $a=0$, $\alpha=0$, $d=d3$, $\theta=0$

---

You can see some guidelines here.