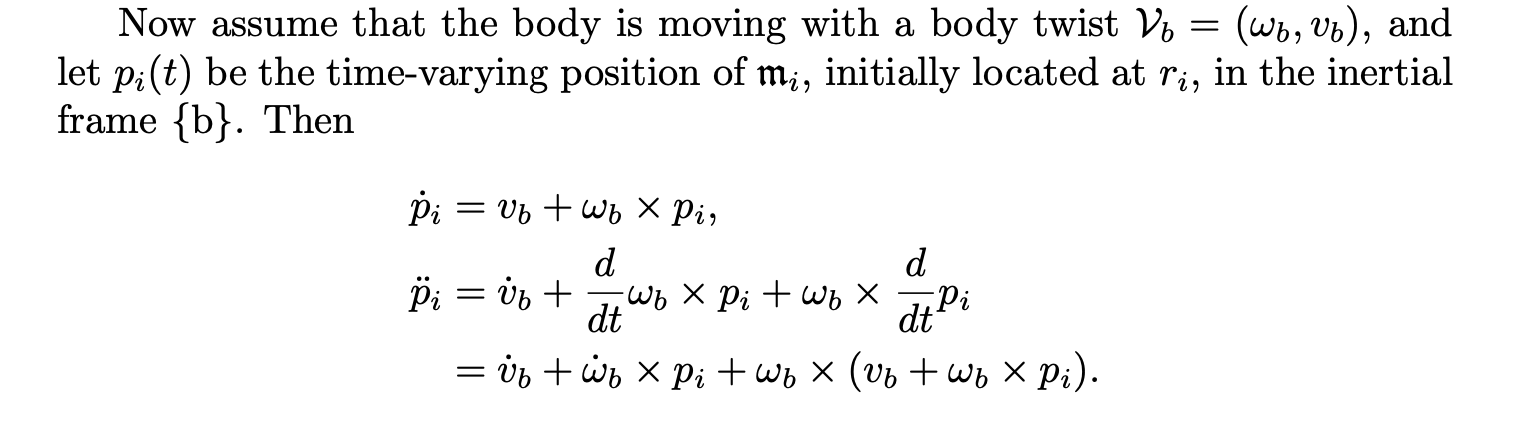0
$\begingroup$

In Park and Lynch's "Modern Robotics" textbook (Section 8.2.1), they give the following derivation for the velocity and acceleration of a point $p_i$ with respect to a fixed body frame $\{b\}$, with the origin of $\{b\}$ at the center of mass of the moving body:

enter image description here

I don't follow the derivation. Typically in introductory dynamics textbooks, the coriolis acceleration of a point in a moving reference frame is given as $2\omega \times v_{rel}$, where $v_{rel}$ is the velocity of the point relative to the moving reference. However, in "Modern Robotics", the point $p_i$ is rigidly fixed to the body and so there is no relative velocity which would give rise to the coriolis acceleration. Also, even if I'm mistaken above, it is not clear to me why the coriolis acceleration term in Park/Lynch is off by a factor of 2 relative to the typical coriolis acceleration term.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

The section title is "8.2 Dynamics of a Single Rigid Body" and you're correct there are no corliosis forces involved. That section is starting from the most basic motion equations where there are no external forces considered in

pi = vb + ωb × pi

The vb is the motion of the center of mass of the rigid body in the inertial frame and the ωb × pi is the cross product that gives you the velocity of the randomly placed point mass (mi) caused by the rotation of the body. Think of a ball rolling on ground at 1 m/s. That is vb = 1. Now pick a point on the surface of the ball such that the point touches the ground the once per revolution. Once per revolution that point reaches reaches 0 m/s (when on ground) and 2 m/s (when it's at the top). That is ωb × pi and will take values between -1 and 1 in the direction of rolling as time changes (remember that pi(t) is the time-varying position of mi). In our ball example ωb × pi will always be zero in the axis orthogonal to the rolling and gravity axes.

This section is purely motion based (location, velocity, and acceleration). Don't confuse it with the coriolis effects. That comes later. I don't understand why author used {b} for both the body frame and inertial frame. I wonder if that is a typo.

Improve this answer
$\endgroup$
1
  • $\begingroup$ The book defines $\{b\}$ as an inertial frame that is instantaneously coincident with the center of mass of the moving body, so this is not a typo. Also, your answer discussed the derivation for $\dot{p_i}$, which makes sense to me, but does not get into the derivation of $\ddot{p_i}$. Here there does seem to be a coriolis force (or something which looks very much like it) involved, namely the term $\omega_b \times v_b$. $\endgroup$
    – Molecular Lionel
    Commented Nov 21, 2023 at 14:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.